Posted on

Shining Light on the Higgs Boson

Figure 1: Here we give a depiction of shining light on monsieur Higgs boson as well as demonstrate the extent of my french.
Figure 1: Here we give a depiction of shining light on monsieur Higgs boson as well as demonstrate the extent of my french.

Hello Particle Nibblers,

This is my first Particlebites entry (and first ever attempt at a blog!) so you will have to bear with me =).

As I am sure you know by now, the Higgs boson has been discovered at the Large Hadron Collider (LHC). As you also may know, `discovering’ a Higgs boson is not so easy since a Higgs doesn’t just `sit there’ in a detector. Once it is produced at the LHC it very quickly decays (in about 1.6 \times 10^{-22} seconds) into other particles of the Standard Model. For us to `see’ it we must detect these particles into which decays. The decay I want to focus on here is the Higgs boson decay to a pair of photons, which are the spin-1 particles which make up light and mediate the electromagnetic force. By studying its decays to photons we are literally shining light on the Higgs boson (see Figure 1)!

The decay to photons is one of the Higgs’ most precisely measured decay channels. Thus, even though the Higgs only decays to photons about 0.2 % of the time, this was nevertheless one of the first channels the Higgs was discovered in at the LHC. Of course other processes (which we call backgrounds) in the Standard Model can mimic the decays of a Higgs boson, so to see the Higgs we have to look for `bumps’ over these backgrounds (see Figure 2). By carefully reconstructing this `bump’, the Higgs decays to photons also allows us to reconstruct the Higgs mass (about 125 GeV in particle physics units or about 2.2 \times 10^{-22} kg in `real world’ units).

Figure 2: Here we show the Higgs `bump' in the invariant mass spectrum of the Higgs decay to a pair of photons.
Figure 2: Here we show the Higgs `bump’ in the invariant mass spectrum of the Higgs decay to a pair of photons.

Furthermore, using arguments based on angular momentum the Higgs decay to photons also allows us to determine that the Higgs boson must be a spin-0 particle which we call a scalar ^1. So we see that just in this one decay channel a great deal of information about the Higgs boson can be inferred.

Now I know what you’re thinking…But photons only `talk to’ particles which carry electric charge and the Higgs is electrically neutral!! And even crazier, the Higgs only `talks to’ particles with mass and photons are massless!!! This is blasphemy!!! What sort of voodoo magic is occurring here which allows the Higgs boson to decay to photons?

The resolution of this puzzle lies in the subtle properties of quantum field theory. More specifically the Higgs can decay to photons via electrically charged `virtual particles‘ ^2. For present purposes its enough to say (with a little hand-waiving) that since the Higgs can talk to the massive electrically charged particles in the Standard Model, like the W boson or top quark, which in turn can `talk to’ photons, this allows the Higgs to indirectly interact with photons despite the fact that they are massless and the Higgs is neutral. In fact any charged and massive particles which exist will in principle contribute to the indirect interaction between the Higgs boson and photons. Crucially this includes even charged particles which may exist beyond the Standard Model and which have yet to be discovered due to their large mass. The sum total of these contributions from all possible charged and massive particles which contribute to the Higgs decay to photons is represented by the `blob’ in Figure 3.

Figure 3: Here we show how the Higgs decays to a pair of photons via `virtual charged particles' or more accurately disturbances in the quantum fields associated with these charge particles.
Figure 3: Here we show how the Higgs decays to a pair of photons via `virtual charged particles’ (or more accurately disturbances in the quantum fields associated with these charge particles)  represented by the grey`blob’.

It is exciting and interesting to think that new exotic charged particles could be hiding in the `blob’ which creates this interaction between the Higgs boson and photons. These particles might be associated with supersymmetry, extra dimensions, or a host of other exciting possibilities. So while it remains to be seen which, if any, of the beyond the Standard Model possibilities (please let there be something!) the LHC will uncover, it is fascinating to think about what can be learned by shining a little light on the Higgs boson!

 

Footnotes:

1. There is a possible exception to this if the Higgs is a spin-2 particle, but various theoretical arguments as well as other Higgs data make this scenario highly unlikely.

2. Note, virtual particle is unfortunately a misleading term since these are not really particles at all (really they are disturbances in their associated quantum fields), but I will avoid going down this rabbit hole for the time being and save it for a later post. See the previous `virtual particles’ link for a great and more in-depth discussion which takes you a little deeper into the rabbit hole.

Further Reading:

http://arxiv.org/abs/0910.4182

http://arxiv.org/abs/1206.1082

http://www.amazon.com/Introduction-Elementary-Particles-David-Griffiths/dp/3527406018/ref=sr_1_4?ie=UTF8&qid=1427166136&sr=8-4&keywords=particle+physics

http://www.amazon.com/Introduction-Quantum-Theory-Frontiers-Physics/dp/0201503972/ref=sr_1_1?ie=UTF8&qid=1427166621&sr=8-1&keywords=peskin+quantum+field+theory

Posted on

Dark Matter in Dwarf Galaxies

Hi Particle Biters,

Last week there were some exciting new results from the Fermi-LAT collaboration in dark matter searches! Dark matter is an exciting topic – we believe that 85% of the known matter in the universe is stuff that we can’t see. “Dark matter” itself is a very broad idea. I’ll need to start by making some assumptions on the kind of dark matter we’re looking for. We assume a dark matter candidate exists as a particle that is both massive and interacts on the scale of the “weak” force (specifically a Weakly Interacting Massive Particle – WIMP). There are lots of reasons that motivate this type of a candidate (cosmic microwave background, baryon acoustic oscillations, large scale structure, gravitational lensing, and galactic rotation curves to name a few), and I can elaborate more in another post if readers are interested. For now, just trust me when I say WIMPs are a well motivated dark matter candidate.

Based on things like galactic rotation curves we can guess the distribution of dark matter in our galaxy – and the highest concentration is in the very center. The problem with looking fimg-thingor dark matter in the center of the galaxy is that there are lots of backgrounds. First, there are ~8 kilo-parsecs worth of spiral arms in between us and the center. That’s a lot of stuff to try to understand. Plus there is a super massive black hole – Sagittarius A* – and an unknown number of pulsars (for example) also in the region of the galactic center. Other good, less chaotic, places to search for WIMPs are small, old satellite galaxies orbiting the Milky Way. One class of these types of galaxies is called dwarf spheroidal galaxies (dsphs) (not to be confused with Disney-type dwarfs). They don’t have enough visible matter to be gravitationally bound (even though they are!), so we know that there must be a high concentration of dark matter in these systems. Below is a picture of the location of some known dsphs. In total about 25 are known that Fermi-LAT will analyze. (you can see Flip’s post for more info too!)

map_milky_way_1000_1000_0

Many of these dsphs were discovered by the Sloan Digital Sky Survey (SDSS). SDSS is a 2.5 m optical telescope located at Apache Point Observatory in New Mexico. It has been surveying the northern sky since 2000. The Fermi-LAT collaboration then points at the location of these dsphs and looks for gamma-rays (high energy photons) which would indicate dark matter annihilation. Since these are old systems, there shouldn’t be any gamma-ray emission from these targets that isn’t from dark matter.

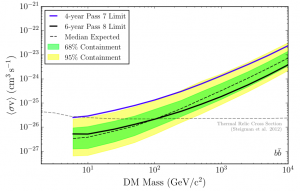Fermi-DMdsphs-P8The Fermi-LAT collaboration has submitted the newest searches for these known dsphs in a paper on arXiv on March 9th. They used 6 years of data and the newest, best event analysis and reconstruction (called Pass 8). The results are on the left: the y-axis shows the cross section times the thermally averaged velocity that we are sensitive to and the x-axis shows different dark matter masses. The blue line shows the previous results obtained by Fermi-LAT. The dashed black line shows what we would expect to see given a specific model of dark matter. In this case we assume that the dark matter decays into b-quarks and anti-b quarks and then from then gamma rays are produced. The solid black line shows the limit of what we actually observed. (Unfortunately no discovery!! That would look like a sharp divergence from the expectation).

Although we haven’t found an indication of dark matter annihilation, we are just becoming sensitive to the “thermal relic” (or the amount of dark matter expected after the big bang shown in the dashed gray line ). So the next few years of these searches are going to be very exciting. I’ll also hint at a future post… there is currently another collaboration (the Dark Energy Survey, or DES), which similarly to SDSS will find more dsphs for us to use as targets – which will only improve our sensitivity. In the next post I’ll talk about these results.

I hope you’ve enjoyed this post! Please post any questions/comments.

Regina

Posted on

Muon to electron conversion

Presenting: Section 3.2 of “Charged Lepton Flavor Violation: An Experimenter’s Guide”
Authors: R. Bernstein, P. Cooper
Reference: 1307.5787 (Phys. Rept. 532 (2013) 27)

Not all searches for new physics involve colliding protons at the the highest human-made energies. An alternate approach is to look for deviations in ultra-rare events at low energies. These deviations may be the quantum footprints of new, much heavier particles. In this bite, we’ll focus on the decay of a muon to an electron in the presence of a heavy atom.

Muons decay
Muons conversion into an electron in the presence of an atom, aluminum.

The muon is a heavy version of the electron.There  are a few properties that make muons nice systems for precision measurements:

  1. They’re easy to produce. When you smash protons into a dense target, like tungsten, you get lots of light hadrons—among them, the charged pions. These charged pions decay into muons, which one can then collect by bending their trajectories with magnetic fields. (Puzzle: why don’t pions decay into electrons? Answer below.)
  2. They can replace electrons in atoms.  If you point this beam of muons into a target, then some of the muons will replace electrons in the target’s atoms. This is very nice because these “muonic atoms” are described by non-relativistic quantum mechanics with the electron mass replaced with ~100 MeV. (Muonic hydrogen was previous mentioned in this bite on the proton radius problem.)
  3. They decay, and the decay products always include an electron that can be detected.  In vacuum it will decay into an electron and two neutrinos through the weak force, analogous to beta decay.
  4. These decays are sensitive to virtual effects. You don’t need to directly create a new particle in order to see its effects. Potential new particles are constrained to be very heavy to explain their non-observation at the LHC. However, even these heavy particles can leave an  imprint on muon decay through ‘virtual effects’ according (roughly) to the Heisenberg uncertainty principle: you can quantum mechanically violate energy conservation, but only for very short times.
Reach of muon conversion experiments from 1303.4097. The y axis is the energy scale that can be probed, the x axis parameterizes how new physics is spread between different CLFV parameters.
Reach of muon conversion experiments from 1303.4097. The y axis is the energy scale that can be probed and the x axis parameterizes different ways that lepton flavor violation can appear in a theory.

One should be surprised that muon conversion is even possible. The process \mu \to e cannot occur in vacuum because it cannot simultaneously conserve energy and momentum. (Puzzle: why is this true? Answer below.) However, this process is allowed in the presence of a heavy nucleus that can absorb the additional momentum, as shown in the comic at the top of this post.

Muon  conversion experiments exploit this by forming muonic atoms in the 1s state and waiting for the muon to convert into an electron which can then be detected. The upside is that all electrons from conversion have a fixed energy because they all come from the same initial state: 1s muonic aluminum at rest in the lab frame. This is in contrast with more common muon decay modes which involve two neutrinos and an electron; because this is a multibody final state, there is a smooth distribution of electron energies. This feature allows physicists to distinguish between the \mu \to e conversion versus the more frequent muon decay \mu \to e \nu_\mu \bar \nu_e in orbit or muon capture by the nucleus (similar to electron capture).

The Standard Model prediction for this rate is miniscule—it’s weighted by powers of the neutrino to the W boson mass ratio  (Puzzle: how does one see this? Answer below.). In fact, the current experimental bound on muon conversion comes from the Sindrum II experiment  looking at muonic gold which constrains the relative rate of muon conversion to muon capture by the gold nucleus to be less than 7 \times 10^{-13}. This, in turn, constrains models of new physics that predict some level of charged lepton flavor violation—that is, processes that change the flavor of a charged lepton, say going from muons to electrons.

The plot on the right shows the energy scales that are indirectly probed by upcoming muonic aluminum experiments: the Mu2e experiment at Fermilab and the COMET experiment at J-PARC. The blue lines show bounds from another rare muon decay: muons decaying into an electron and photon. The black solid lines show the reach for muon conversion in muonic aluminum. The dashed lines correspond to different experimental sensitivities (capture rates for conversion, branching ratios for decay with a photon). Note that the energy scales probed can reach 1-10 PeV—that’s 1000-10,000 TeV—much higher than the energy scales direclty probed by the LHC! In this way, flavor experiments and high energy experiments are complimentary searches for new physics.

These “next generation” muon conversion experiments are currently under construction and promise to push the intensity frontier in conjunction with the LHC’s energy frontier.

 

 

Solutions to exercises:

  1. Why do pions decay into muons and not electrons? [Note: this requires some background in undergraduate-level particle physics.] One might expect that if a charged pion can decay into a muon and a neutrino, then it should also go into an electron and a neutrino. In fact, the latter should dominate since there’s much more phase space. However, the matrix element requires a virtual W boson exchange and thus depends on an [axial] vector current. The only vector available from the pion system is its 4-momentum. By momentum conservation this is $p_\pi = p_\mu + p_\nu$. The lepton momenta then contract with Dirac matrices on the leptonic current to give a dominant piece proportional to the lepton mass. Thus the amplitude for charged pion decay into a muon is much larger than the amplitude for decay into an electron.
  2. Why can’t a muon decay into an electron in vacuum? The process \mu \to e cannot simultaneously conserve energy and momentum. This is simplest to see in the reference frame where the muon is at rest. Momentum conservation requires the electron to also be at rest. However, a particle has rest energy equal to its mass, but now there’s now way a muon at rest can pass on all of its energy to an electron at rest.
  3. Why is muon conversion in the Standard Model suppressed by the ration of the neutrino to W masses? This can be seen by drawing the Feynman diagram (fig below from 1401.6077). Flavor violation in the Standard Model requires a W boson. Because the W is much heavier than the muon, this must be virtual and appear only as an internal leg. Further, W‘s couple charged leptons to neutrinos, so there must also be a virtual neutrino. The evaluation of this diagram into an amplitude gives factors of the neutrino mass in the numerator (required for the fermion chirality flip) and the W mass in the denominator. For some details, see this post.
    Screen Shot 2015-03-05 at 4.08.58 PM

Further Reading:

  • 1205.2671: Fundamental Physics at the Intensity Frontier (section 3.2.2)
  • 1401.6077: Snowmass 2013 Report, Intensity Frontier chapter

 

 

 

Posted on

LHC Run II: What To Look Out For

The Large Hadron Collider is the world’s largest proton collider, and in a mere five years of active data acquisition, it has already achieved fame for the discovery of the elusive Higgs Boson in 2012. Though the LHC is currently off to allow for a series of repairs and upgrades, it is scheduled to begin running again within the month, this time with a proton collision energy of 13 TeV. This is nearly double the previous run energy of 8 TeV,  opening the door to a host of new particle productions and processes. Many physicists are keeping their fingers crossed that another big discovery is right around the corner. Here are a few specific things that will be important in Run II.

 

1. Luminosity scaling

Though this is a very general category, it is a huge component of the Run II excitement. This is simply due to the scaling of luminosity with collision energy, which gives a remarkable increase in discovery potential for the energy increase.

If you’re not familiar, luminosity is the number of events per unit time and cross sectional area. Integrated luminosity sums this instantaneous value over time, giving a metric in the units of 1/area.

lumi                          intLumi

 In the particle physics world, luminosities are measured in inverse femtobarns, where 1 fb-1 = 1/(10-43 m2). Each of the two main detectors at CERN, ATLAS and CMS, collected 30 fb-1 by the end of 2012. The main point is that more luminosity means more events in which to search for new physics.

Figure 1 shows the ratios of LHC luminosities for 7 vs. 8 TeV, and again for 13 vs. 8 TeV. Since the plot is in log scale on the y axis, it’s easy to tell that 13 to 8 TeV is a very large ratio. In fact, 100 fb-1 at 8 TeV is the equivalent of 1 fb-1 at 13 TeV. So increasing the energy by a factor less than 2 increase the integrated luminosity by a factor of 100! This means that even in the first few months of running at 13 TeV, there will be a huge amount of data available for analysis, leading to the likely release of many analyses shortly after the beginning of data acquisition.

lumiRatio
Figure 1: Parton luminosity ratios, from J. Stirling at Imperial College London (see references.)

 

2. Supersymmetry

Supersymmetry theory proposes the existence of a superpartner for every particle in the Standard Model, effectively doubling the number of fundamental particles in the universe. This helps to answer many questions in particle physics, namely the question of where the particle masses came from, known as the ‘hierarchy’ problem (see the further reading list for some good explanations.)

Current mass limits on many supersymmetric particles are getting pretty high, concerning some physicists about the feasibility of finding evidence for SUSY. Many of these particles have already been excluded for masses below the order of a TeV, making it very difficult to create them with the LHC as is. While there is talk of another LHC upgrade to achieve energies even higher than 14 TeV, for now the SUSY searches will have to make use of the energy that is available.

SUSYxsec
Figure 2: Cross sections for the case of equal degenerate squark and gluino masses as a function of mass at √s = 13 TeV, from 1407.5066. q stands for quark, g stands for gluino, and t stands for stop.

 

Figure 2 shows the cross sections for various supersymmetric particle pair production, including squark (the supersymmetric top quark) and gluino (the supersymmetric gluon). Given the luminosity scaling described previously, these cross sections tell us that with only 1 fb-1, physicists will be able to surpass the existing sensitivity for these supersymmetric processes. As a result, there will be a rush of searches being performed in a very short time after the run begins.

 

3. Dark Matter

Dark matter is one of the greatest mysteries in particle physics to date (see past particlebites posts for more information). It is also one of the most difficult mysteries to solve, since dark matter candidate particles are by definition very weakly interacting. In the LHC, potential dark matter creation is detected as missing transverse energy (MET) in the detector, since the particles do not leave tracks or deposit energy.

One of the best ways to ‘see’ dark matter at the LHC is in signatures with mono-jet or photon signatures; these are jets/photons that do not occur in pairs, but rather occur singly as a result of radiation. Typically these signatures have very high transverse momentum (pT) jets, giving a good primary vertex, and large amounts of MET, making them easier to observe. Figure 3 shows a Feynman diagram of such a decay, with the MET recoiling off a jet or a photon.

feynmanMonoX
Figure 3: Feynman diagram of mono-X searches for dark matter, from “Hunting for the Invisible.”

 

Though the topics in this post will certainly be popular in the next few years at the LHC, they do not even begin to span the huge volume of physics analyses that we can expect to see emerging from Run II data. The next year alone has the potential to be a groundbreaking one, so stay tuned!

 

References: 

Further Reading: